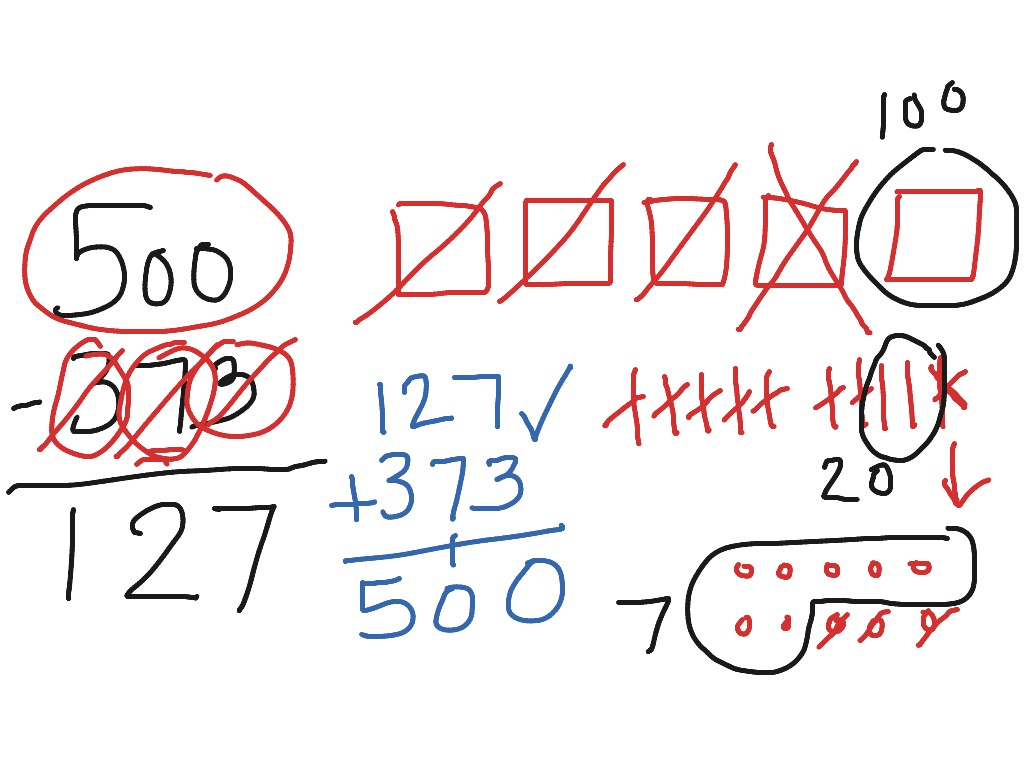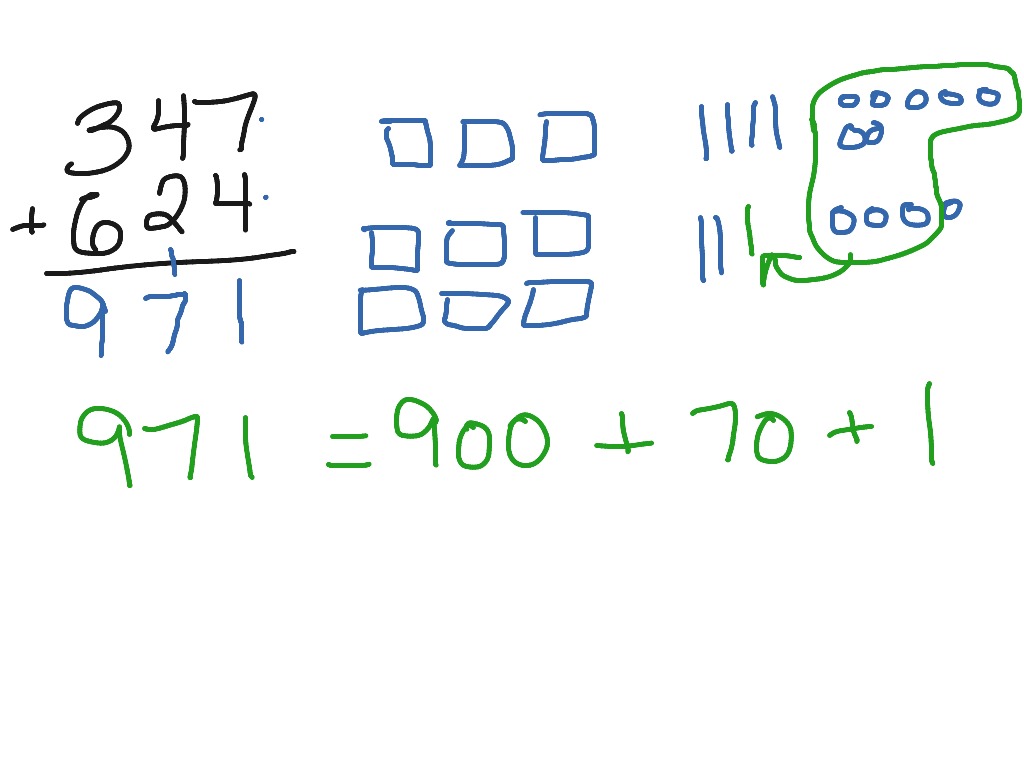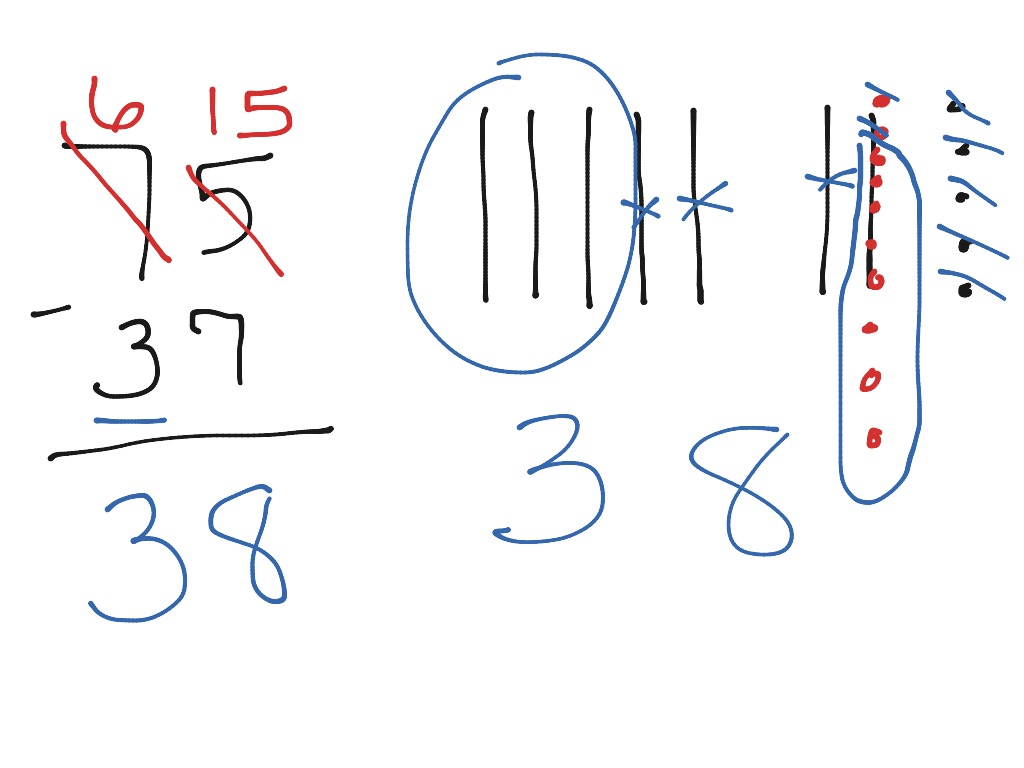Math Proof Drawing
Math Proof Drawing - This is a proof without words. They help build your intuition, allow you to have fun playing around, and sometimes pictures can even. Here’s a proof for you. Web google says alphaproof uses reinforcement learning to prove mathematical statements in the formal language called lean.the system trains itself by generating and verifying. Geometric intuition and pictures allow to prove results visually. Web there are a few reasons math problems that involve advanced reasoning are difficult for ai systems to solve. The math proofs that will be covered in this website fall under the category of basic or introductory proofs. A structured approach by daniel j. These types of problems often require forming and drawing on. Web strictly speaking, q.e.d. (as stated) means something like as was to be shown, so (strictly) it is only appropriate if the last thing in your proof, indeed, was the thing to be. We will show how to use these proof. Web there are a few reasons math problems that involve advanced reasoning are difficult for ai systems to solve. A structured approach by daniel j. This is a proof without words. Here’s a proof for you. Train your ability to do proofs by induction, contradiction and more. Web these are the three addition methods we learned in unit one, lesson five of the math expressions series. Web strictly speaking, q.e.d. (as stated) means something like as was to be shown, so (strictly) it is only appropriate if the last thing in your proof, indeed, was the thing to be. These types of problems often require forming and drawing on. This is a proof without words. Web direct proofs are especially useful when proving implications. Web this video shows how we use proof drawings for subtraction. Web strictly speaking, q.e.d. (as stated) means something like as was to be shown, so (strictly) it is only appropriate if the last thing in your proof, indeed, was the thing to be. Web calculators and convertors for stem, finance, fitness, construction, cooking, and more. These types of problems often require forming and drawing on. A structured approach by daniel j. Ben orlin math october 2, 2019 3 minutes. Here’s a proof for you. Web this article introduces the idea of generic proof for younger children and illustrates how one example can offer a proof of a general result through unpacking its underlying structure. The general format to prove p → q is this: Here’s a proof for you. Web direct proofs are especially useful when proving implications. Web strictly speaking, q.e.d. (as stated) means something like as was to be shown, so (strictly) it is only appropriate if the last thing in your proof, indeed, was the thing to be. This is a proof without words. The math proofs that will be covered. The argument may use other. Geometric intuition and pictures allow to prove results visually. In this set of notes, we explore basic proof techniques, and how they can be understood by a grounding in propositional logic. Web after months of rigorous training, the students sat for two exams, three problems per day — in algebra, combinatorics, geometry and number theory.. The kids ungroup rather than borrow. Train your ability to do proofs by induction, contradiction and more. Geometric intuition and pictures allow to prove results visually. The argument may use other. Web google says alphaproof uses reinforcement learning to prove mathematical statements in the formal language called lean.the system trains itself by generating and verifying. Web math 22a unit 6: Web this article introduces the idea of generic proof for younger children and illustrates how one example can offer a proof of a general result through unpacking its underlying structure. The general format to prove p → q is this: Web direct proofs are especially useful when proving implications. What formula does figure (3). The general format to prove p → q is this: These types of problems often require forming and drawing on. Web after months of rigorous training, the students sat for two exams, three problems per day — in algebra, combinatorics, geometry and number theory. A structured approach by daniel j. Erin dunbar 10 years ago. Web this article introduces the idea of generic proof for younger children and illustrates how one example can offer a proof of a general result through unpacking its underlying structure. Web using the new groups above method and using a proof drawing with regrouping. The math proofs that will be covered in this website fall under the category of basic. The argument may use other. Web these are the three addition methods we learned in unit one, lesson five of the math expressions series. Web how to prove it: Ben orlin math october 2, 2019 3 minutes. We will show how to use these proof. A structured approach by daniel j. We will show how to use these proof. Ben orlin math october 2, 2019 3 minutes. What formula does figure (3). Web how to prove it: Web direct proofs are especially useful when proving implications. Web these are the three addition methods we learned in unit one, lesson five of the math expressions series. Web math 22a unit 6: In this set of notes, we explore basic proof techniques, and how they can be understood by a grounding in propositional logic. Erin dunbar 10 years ago. Drawing pictures is incredibly useful when doing maths. Web how to prove it: Web this video shows how we use proof drawings for subtraction. Erin dunbar 10 years ago. Web this article introduces the idea of generic proof for younger children and illustrates how one example can offer a proof of a general result through unpacking its underlying structure. How to write a proof. Synthesizing definitions, intuitions, and conventions. Web this video shows how we use proof drawings for subtraction. Working with odd and even numbers. Web this article introduces the idea of generic proof for younger children and illustrates how one example can offer a proof of a general result through unpacking its underlying structure. The general format to prove p → q is this: What formula does figure (3). Web strictly speaking, q.e.d. (as stated) means something like as was to be shown, so (strictly) it is only appropriate if the last thing in your proof, indeed, was the thing to be. Web after months of rigorous training, the students sat for two exams, three problems per day — in algebra, combinatorics, geometry and number theory. The math proofs that will be covered in this website fall under the category of basic or introductory proofs. See the other videos for subtracting across zeros, or for other methods of. Geometric intuition and pictures allow to prove results visually. Web google says alphaproof uses reinforcement learning to prove mathematical statements in the formal language called lean.the system trains itself by generating and verifying. Here’s a proof for you. Erin dunbar 10 years ago. These types of problems often require forming and drawing on.What Is A Proof Drawing In Math Drawing.rjuuc.edu.np
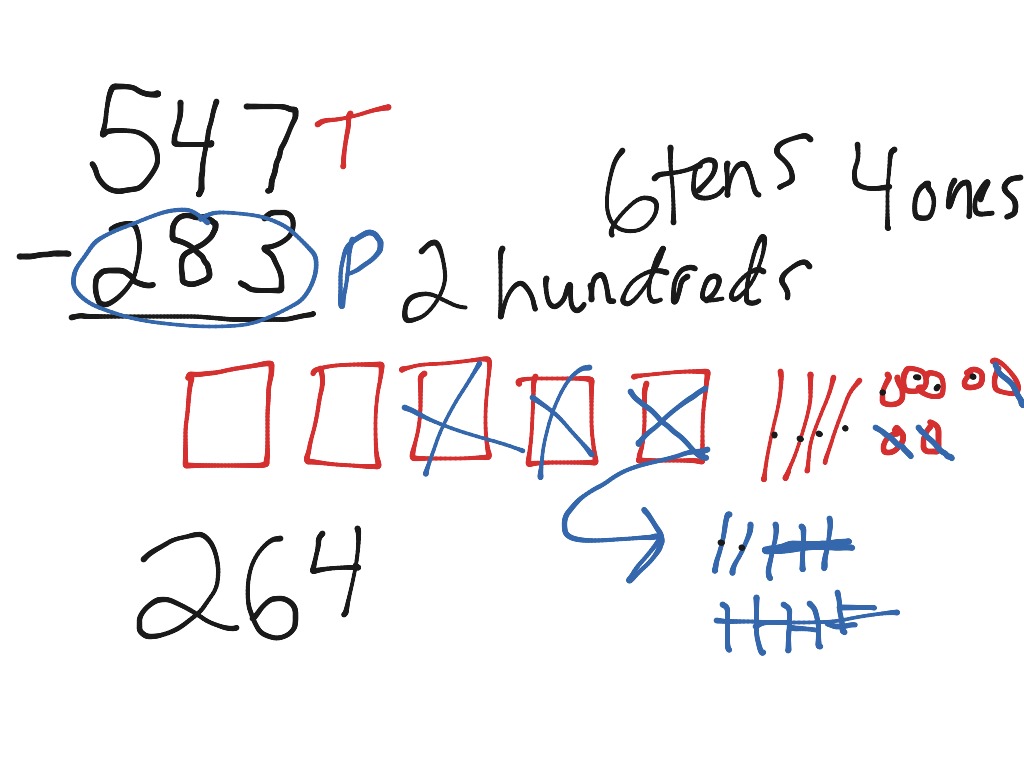
3Digit subtraction using Proof Drawings Math, Elementary Math, 2nd
Proof Drawing Math Ellipse Geometric Construction Besant Definition
Subtracting proof drawing Math, subtraction ShowMe
Math Subtraction Proof Drawing YouTube
Proof Drawing Math 2Nd Grade Warehouse of Ideas
Third gradeunit 4 adding with proof drawings Math, Elementary Math
How to make a proof drawing math strategy YouTube
Proof drawing for subtraction Math, Elementary Math, 2nd Grade Math
What Is A Proof Drawing Math 2nd Grade Homey Gears
The Argument May Use Other.
Geometric Intuition And Pictures Allow To Prove Results Visually.
Web Using The New Groups Above Method And Using A Proof Drawing With Regrouping.
Web Calculators And Convertors For Stem, Finance, Fitness, Construction, Cooking, And More.
Related Post: